Understanding How Power Factor is Calculated
How Power Factor is Calculated - Calculating PF is crucial for understanding the efficiency of power use in AC circuits. In electrical systems, power factor (PF) represents the ratio between real power, which performs actual work, and apparent power (S), which is the total power supplied. A precise PF calculation can help optimize energy use, reduce costs, and enhance overall system performance.
Power Quality Analysis Training
Request a Free Power Quality Training Quotation
Understanding PF Components
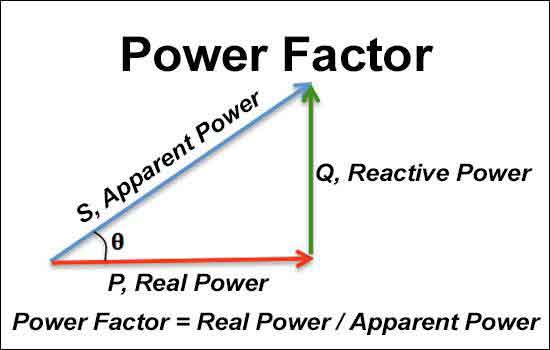
To calculate PF, it’s essential first to understand the types of power involved: real power (active power), otherwise known as "P", reactive power (Q), and apparent power (S). Real power, measured in kilowatts (kW), is the power that does useful work. Reactive power, measured in kilovolt-amperes reactive (kVAR), is necessary for creating and sustaining magnetic fields, especially with inductive loads like motors or capacitive loads such as capacitors. Apparent power, expressed in kilovolt-amperes (kVA), combines both real and reactive power.
PF is the ratio of P to S, indicating how efficiently the electrical energy is used. The formula for PF is:
PF = P (kW) / S (kVA)
This ratio shows how much of the S is effectively converted into useful work, with a PF of 1 indicating perfect efficiency.
The Process of Calculating PF
To calculate PF, you need measurements of both P and S. An analyzer or quality analyzer is typically used in electrical systems to capture these values accurately.
Measure Real Power (kW): This is the actual power consumed by the system to perform work, like running machinery or lighting. Real power is what you aim to maximize within the limits of efficiency.
Measure Apparent Power (kVA): S is the product of the circuit’s voltage (V) and current (A). The equation for S in an AC circuit is:
kVA = Voltage (V) × Current (A)
Calculate the PF: With P and S measured, PF can be calculated by dividing P by S:
PF = kW / kVA
Consider Phase Angle and Q:
In AC circuits, the phase angle between current and voltage affects the PF. Inductive loads, which create a lagging PF, result in a larger phase angle, increasing reactive power. This reactive power doesn’t perform useful work but influences the PF calculation by affecting the total apparent power.
Why Power Factor Calculation Matters
A poor PF means higher S consumption, which can lead to inefficiencies and increased operational costs. Understanding how PF is calculated allows companies to implement PF correction measures, typically by adding capacitive loads to balance inductive loads. PF correction reduces the need for Q, thereby improving the ratio of real power to apparent power.
Improving Power Factor in Electrical Systems
Investing in PQ analyzers and implementing PF correction can help maintain a high PF, ensuring efficient usage and reducing costs. Proper correction methods, such as adding capacitors to counterbalance inductive loads, help improve PF and lower the overall electrical consumption.
Calculating PF accurately is essential for optimizing electricity consumption in AC circuits. By focusing on the ratio of P to S and understanding the effects of Q and phase angle, businesses can achieve more efficient energy use and reduce costs associated with poor PF.
