Equivalent Resistance

Static electricity is a fascinating phenomenon that can be both awe-inspiring and dangerous. Understanding its basic concepts, including the movement of charged particles and the causes of positive and negative charge imbalance, is crucial in preventing potential hazards. By taking preventive measures such as grounding equipment and surfaces, using anti-static materials, and increasing humidity, we can avoid the buildup of static charges and protect ourselves and our environment from its hazards. It is also important to understand the potential dangers and take appropriate precautions, particularly when working with flammable or explosive materials. With this knowledge, we can safely harness the power and explore its many fascinating properties.

What is static electricity?

It results from the movement of charged particles, called electrons and particles called protons, which make up the atoms of matter. Atoms are made up of protons, neutrons, and electrons. Protons have a positive charge, electrons have a negative charge, and neutrons have no charge. In most atoms, the positive charge of the protons is balanced by the negative charge of the electrons, resulting in a neutral object.

However, when two objects come in contact, the extra electrons from one object may transfer to the other, creating a charge imbalance. This results in one object having a positive charge and the other having a negative electric charge. These charges repel, and when the objects are separated, they retain the charges, creating a static charge.

What causes static electricity?

As previously mentioned, it is caused by the movement of charged particles. When there is a charge imbalance between two objects, it can create a static charge. This can occur through friction, conduction, or induction.

Friction occurs when two objects rub against each other, causing electrons to transfer from one object to the other. For example, this is why you may experience it when you rub a balloon against your hair, causing your hair stand to stand up.

Conduction occurs when two objects come in contact and transfer electrons between them. For example, when you touch a doorknob, you may receive a static shock if the doorknob is charged.

Induction occurs when a charged object is brought near a neutral object, causing a charge to be induced in the neutral object.

How does static electricity work?

It creates a charge imbalance between two objects, resulting in one object having a positive charge and the other having a negative charge. These charges repel each other, and when the objects are separated, they retain the charges, creating a static charge.

When a charged object comes in contact with a neutral object, the charge may transfer, resulting in a static shock. This occurs when the charge imbalance is released as a spark. Static shocks are usually harmless but can sometimes be dangerous, particularly in hazardous environments.

Can static electricity hurt you?

While it is usually harmless, it can sometimes be dangerous. A static shock occurs when a charged object comes in contact with a person or object that is not charged. The shock results from the charge imbalance being released as a spark. The shock can be uncomfortable, and in rare cases, it can cause serious injury, mainly if it occurs in a hazardous environment, such as around flammable materials.

What are the dangers of static electricity?

It can be dangerous in certain situations, such as when working with flammable or explosive materials. A spark from it can ignite flammable gases or vapours, leading to fires or explosions. In industrial settings, it is important to take precautions to prevent the buildup of static charges and to discharge any charges that may occur.

How do you discharge static electricity?

There are several ways to discharge it, including:

• Grounding: Connecting the charged object to a ground source such as the earth, which dissipates the excess charge.
• Anti-static materials: Wearing clothes made of anti-static materials or using anti-static sprays can prevent the buildup of static charges.
• Humidification: Increasing the humidity in a room can help reduce a buildup by adding moisture to the air.
• Use of ionizers: Ionizers can neutralize it by releasing negative ions into the air.
• Proper grounding of equipment: Ensuring that all equipment and surfaces are properly grounded can prevent the buildup of static charges.

How can you prevent static electricity?

Preventing buildup involves taking several steps, such as:

• Using anti-static materials: Clothing made of anti-static materials or anti-static sprays can prevent the buildup of static charges.
• Grounding: Ensuring that all equipment and surfaces are properly grounded can prevent the buildup of static charges.
• Humidification: Increasing the humidity in a room can help reduce a buildup by adding moisture to the air.
• Proper handling of flammable materials: When working with flammable or explosive materials, it is important to take proper precautions to prevent a buildup and discharge of any charges.
• Using equipment designed for use in hazardous environments: Equipment designed for use in hazardous environments is designed to prevent a buildup and sparks from occurring.

In addition to these preventive measures, it is important to understand the basics of static electricity and the factors that contribute to its buildup. This knowledge can help individuals take steps to avoid buildup and prevent any potential hazards.

Van de Graaff Generator: An Experiment with Static Electricity

One fascinating example of this is the Van de Graaff generator. This device was invented in 1929 by Robert J. Van de Graaff and is used to generate high voltages of static electricity. The generator transfers electrons from a moving belt to a metal sphere, creating a charge imbalance between the two objects.

This charge imbalance creates a strong electric field, which can be used to demonstrate various phenomena. For example, the electric field can cause hairs on a person's head or arm to stand up due to the repulsion between the positively charged hair and the positively charged metal sphere.

Equivalent resistance is the total resistance of a circuit that simplifies multiple resistors into a single resistor. It is calculated differently for series and parallel circuits, helping determine current flow, voltage distribution, and overall power consumption in electrical networks.

What is Equivalent Resistance?

Equivalent resistance is a crucial concept for individuals working with electrical circuits. It: 

✅ Represents the single resistance that replaces multiple resistors in a circuit.

✅ Simplifies calculations of current, voltage, and power.

✅ Found using series or parallel formulas based on resistor arrangement.

By mastering the calculation methods for series and parallel circuits, understanding the relationship with Ohm's law, and applying Kirchhoff's laws in more complex situations, you can efficiently analyze and design electrical systems that meet the requirements of various applications. Furthermore, by incorporating equivalent resistance calculations into your skillset, you'll be better prepared to tackle the exciting challenges presented by the ever-evolving world of electronics and power systems.

Equivalent Resistance in Series vs. Parallel Circuits

To calculate the equivalent resistance of a network, it is often necessary to determine the sum of the individual resistances. This involves adding the resistance values of each individual resistor in the network, providing a baseline for further calculations. This is a resistance formula for a single resistor, as well as for calculating resistors connected in parallel or in series.

Electrical resistance (R) is a measure of the opposition to the flow of electric current within a circuit. It is determined by the material and dimensions of the conductor, as well as the temperature and other environmental factors. It is measured in ohms (Ω), with lower R indicating greater conductivity and vice versa.

Equivalent Resistance Formula

When a group of resistors is connected end-to-end, they form a series. In a series circuit, the total resistance is equal to the sum of the individual Rs, as current must flow through each resistor in turn. The equivalent resistance of a series circuit is calculated by adding the values of each individual resistor:

Req = R1 + R2 + R3 + ...

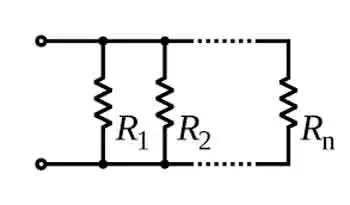
Resistors connected across the same potential difference, or applied voltage, are referred to as being connected in parallel. In a parallel circuit, the total R is less than the R of any individual resistor as the current divides among the resistors. The equivalent resistance of a parallel circuit is calculated using the reciprocal formula:

1/Req = 1/R1 + 1/R2 + 1/R3 + ...

Equivalent resistance is the single R value that can replace a group of resistors in a circuit, offering the same overall R. This simplifies circuit analysis and allows for more straightforward calculations of current and voltage values.

Ohm's law is an essential principle in electronics and relates closely to equivalent resistance. Ohm's law states that the current (I) flowing through a resistor is directly proportional to the applied voltage (V) and inversely proportional to the R. The equation is:

V = IR

By calculating equivalent resistance for a given circuit, we can use Ohm's law to determine the overall current flowing through the circuit and the voltage drops across each resistor.

Series and parallel circuits exhibit different properties when it comes to equivalent resistance. In series circuits, the overall R increases with the addition of more resistors, which means the total current will decrease. In parallel circuits, adding more resistors results in a decrease in total resistance, causing an increase in total current. Understanding these differences is crucial for designing circuits that meet specific electrical requirements.

Kirchhoff's Law

Kirchhoff's laws are another essential tool for calculating equivalent resistance in more complex circuits that cannot be simplified as purely series or parallel. Kirchhoff's current law (KCL) states that the sum of currents entering a junction in a circuit must equal the sum of currents leaving the junction. Kirchhoff's voltage law (KVL) asserts that the sum of the voltages around any closed loop in a circuit must equal zero. By applying these laws, we can create a system of equations to solve for unknown resistance values in a network, ultimately determining the equivalent resistance.

Real-World Applications

Real-world applications of equivalent resistance can be found in various fields, such as electronics, power distribution, and telecommunications. For example, various components have different R values in electronic devices, such as smartphones. Calculating the equivalent resistance enables engineers to design efficient power management systems, ensuring optimal performance and extended battery life. In power distribution networks, equivalent resistance calculations are essential for determining voltage drops and current values across transmission lines, enabling the design of efficient and reliable power delivery systems.

Understanding equivalent resistance is vital for professionals and students who deal with electrical circuits. From simplifying complex networks to designing efficient circuits, equivalent resistance plays a central role in analyzing and developing modern electronic systems. Familiarity with this concept and associated principles like Ohm's law and Kirchhoff's laws ensures a strong foundation for anyone working with electronics, power systems, and telecommunications.

Technology Advances

As technology advances, the importance of equivalent resistance in designing and analyzing new devices and systems will only grow. Calculating and understanding equivalent resistance enables engineers to push the boundaries of innovation and improve upon existing systems, resulting in more efficient, high-performance solutions for consumers and industries alike.

To further develop your understanding of equivalent resistance and related concepts, consider exploring the wealth of educational resources available online and in textbooks, as well as seeking hands-on experience through workshops or real-world projects. Applying this knowledge to practical scenarios will enable you to tackle various electrical and electronic challenges.

Related Articles

• Electricity Fundamentals

• Electricity Questions

• Electricity History

Home electricity powers lighting, appliances, and electronics using energy from the grid or renewable sources like solar. Understanding usage, safety, and efficiency helps reduce costs and improve performance in daily life.

What is Home Electricity?

Home electricity refers to the power used to operate household systems and devices.

✅ Powers lights, appliances, HVAC, and electronics

✅ Supplied by utilities or solar energy systems

✅ Measured in kilowatt-hours and billed monthly

How Electricity Enters and Flows Through Your Home

Electricity originates from a distant power station, fueled by coal, natural gas, nuclear power, or renewable sources, and is transformed into high-voltage energy for long-distance transmission. Along the way, transformers step this voltage down to safe, usable levels. When it reaches your home, the electricity flows through a service line to a main panel, where circuit breakers distribute it to branch circuits that power every room and outlet.

In the United States, most residential circuits provide about 120 volts of alternating current (AC) at 60 Hz. Voltage measures the energy per unit charge: 120 volts means 120 joules of energy are transported by each coulomb of charge. One coulomb per second equals one ampere, so an appliance drawing 1 ampere at 120 volts consumes energy at 120 watts—120 joules per second.

How Appliances Use Electrical Energy

When you switch on an appliance, it allows 120 volts of potential to push current through the device—similar to using water pressure to move water through a pipe. As electric charge flows, energy is transformed into useful work like heating, lighting, or mechanical motion. Voltage gradually drops to nearly zero by the time the current exits the appliance.

This “spent” charge is carried back to your electrical panel via the neutral wire and ultimately to the ground through the grounding system. The ground serves as a vast reservoir, stabilizing voltages and protecting users in the event of a fault.

Understanding Home Wiring and Safety

In a standard polarized receptacle, the smaller prong connects to the hot wire (120 volts), and the wider prong to the neutral (0 volts). The hot wire delivers the current; the neutral completes the circuit back to the panel. A separate ground wire exists for safety—it’s not part of the working circuit but protects against electric shock if a fault occurs.

Most wall outlets contain two receptacles (a duplex) wired in parallel. This means two appliances plugged in will receive the same 120-volt supply but can draw different amounts of current. Parallel wiring is standard throughout the home, allowing consistent voltage distribution while accommodating various electrical loads.

Where the Energy Comes From and Where It Goes

Electricity used at home ultimately comes from generators that elevate the voltage of electric charge before it travels across the grid. After powering your home, the now low-energy charge returns to the ground. This closed-loop system—from the earth to the generator and back—enables efficient energy use and the safe disposal of spent charge.

Whether your electricity comes from traditional utilities or rooftop solar panels, the underlying principles remain the same: raise voltage at the source, deliver it efficiently, use it safely, and return it to ground. Understanding these concepts helps homeowners stay informed, reduce waste, and use electricity more safely and effectively.

Related Articles

• Electricity Fundamentals

• Electricity Questions

• Electricity History

Saving electricity through energy efficiency, power factor correction, load balancing, and demand response integrates smart meters, VFDs, and SCADA to cut losses, optimize voltage, and improve grid reliability in industrial and commercial systems.

What Is Saving Electricity?

Saving electricity applies engineering methods to cut losses, optimize loads, and improve efficiency and reliability.

✅ Implement power factor correction capacitors to reduce reactive power.

✅ Use VFDs for motor speed control and peak demand reduction.

✅ Deploy smart meters, SCADA, and analytics for load balancing.

Saving electricity becomes an increasingly important issue the more the cost of power increases. There is no such thing as cheap electricity. That is a basic fact in today's world of increasing costs for all forms of energy. It's a common fact that vast amounts of electric power are wasted every year in the world, mostly in areas where power is cheap and abundant. However, in recent years, as the supply of electricity generation has remained static and at the same time demand for power continues to increase, the power prices have increased dramatically. For a refresher on the fundamentals, see this brief guide to what electricity is to better frame consumption trends.

One of the leading cost inputs of electric energy is the cost of generation energy inputs. For instance, if all power generation came from oil, you can imagine what the cost of power would be. Since most power is generated from burning coal and natural gas, as the cost of these commodities increases, so does the cost of electric generation. A quick overview of primary sources of electricity clarifies how fuel price volatility feeds into rates.

Saving electricity, on the other hand, is one of the least expensive ways to generate large amounts of power is from the use of water in hydroelectric generation stations. Hydropower is covered among methods that generate electricity efficiently when geography permits.

The other least expensive way to generate power is from the use of uranium in nuclear power stations. Yes, nuclear power stations are very expensive to build but given the amount of power they generate, plus the fact that they operate 24 hours a day, makes them a very cost-effective way to generate electric power. Alongside nuclear, many regions are expanding alternative electricity options to diversify supply.

Using Less, Quickly
Energy conservation can be accomplished quickly when each home saves electricity through less consumption. This is accomplished by turning lights off when not needed. You can also turn off appliances and use less heat. First, power saver improvements can be made to the electrical equipment such that consumers receive the same services but with less power consumed. (These are sometimes called “efficiency improvements”). In the second case, the energy consumer takes deliberate measures to reduce energy use through changes in operations or procedure which may also cause inconvenience and result in a loss of service. These are typically called “behavioral” changes. For step-by-step ideas, practical checklists on how to save electricity can help households prioritize actions without sacrificing comfort.

Technical Efficiency Improvements
The technical measures to save consumption quickly are similar to those used to save power slowly except that they may be implemented with greater intensity by combining them with special subsidies or delivery schemes. Three examples are described below.

Retrofits—especially retrofits of large, energy-using facilities—are attractive targets during an energy crisis. About 8% of industrial power consumption is used to make compressed air, much of which is lost through system leaks. Understanding the relationship between electricity and power helps target leaks and idle loads more effectively.

Lighting replacements have been the most frequently used retrofit strategy. Replacing incandescent light bulbs with compact fluorescents (CFLs) was used in Brazil, California, and New Zealand. California consumers installed nearly eight million CFLs during the crisis period, resulting in almost 500 MW of demand reduction (Pang 2003). California cities replaced millions of traffic lights with LED lamps, each saving about 80 watts. California aggressively encouraged consumers to replace older appliances with new units qualifying for the Energy Star endorsement of high efficiency. While there is no such thing as truly free power, discussions about free electricity often highlight bill-lowering strategies and policy incentives.

When it comes to saving electricity, there are many techniques one can easily apply. Conservation (or using less) is the best and most extreme way. But when using less is not possible, the next best thing for energy conservation is the use of more energy efficient electrical equipment, such as:

• Energy efficient electric Motors. Electric motors consumer 75 per cent of all power consumed by industry and any large consumer of power will save money by installing more energy efficient electric motors.
• Variable frequency drives that operate and control electric motors and are good at saving electricity. These devices control the speed and torque and starting characteristics of large horsepower motors and one of the best ways for using less and earning more.
• Energy efficient electric lighting is also good at saving electricity. There are lots of more efficient electric lighting technologies and control systems on the market today.
• Energy efficient home appliances like refrigerators and dishwashers and clothes washers and dryers can make a large impact on your power bill.
• A High-Efficiency Energy heating system saves heating and money.
• Off-peak electric utility rates from electric utilities reward customers for saving electricity because they can provide consumers with an incentive to shift their power consumption into periods of the day when the electric utility is not at or near full generating capacity like late at night.

Related Articles

• Electricity Fundamentals

• Electricity Questions

• Electricity History

Electricity demand Canada drives load forecasting, grid reliability, peak demand management, renewable integration, and capacity planning across provinces, informing energy consumption trends, transmission constraints, demand response programs, and ISO market operations.

What Is Electricity Demand Canada?

It is the national electrical load across sectors guiding forecasting, grid planning, and reliability.

✅ Provincial load profiles and peak diversity analysis

✅ Demand response and time-of-use optimizing peak loads

✅ Transmission constraints and capacity expansion planning

Electricity Demand in Canada will continue to put, in the longer term, upward pressure on prices for consumers in both regulated and restructured or deregulated markets. For context, market fundamentals and policy design both shape electricity prices across provinces today, influencing bills over time.

These dynamics play out within an integrated electric power system that balances reliability, affordability, and decarbonization goals.

These pricing impacts will be felt due to the cost of developing new generation and transmission facilities in order to replace ageing infrastructure and due to the increased use of renewable sources of generation (e.g., wind, solar, biomass). Continued strength of oil and gas prices are expected to keep electricity prices higher in regions that rely on natural gas and oil products as input fuels for power generation. Understanding the mix of hydro, wind, solar, nuclear, and gas requires a grasp of how electricity is generated across Canada’s grid components.

With the exception of Ontario (due to its large summer seasonal air conditioning requirement for electricity demand, Canada is a winter-peaking system and so the highest demand loads usually occur in the winter. However, for provinces that have competitive wholesale markets (e.g. Alberta and Ontario), near-term price pressures could result from the increase in summer cooling demand. Prices could also experience additional upward pressure from fuels used to generate electricity; namely, oil and natural gas. These pressures could be further supported by unusual weather events, unplanned generation outages and transmission system failures. If wholesale price pressures are to be passed on to consumers, the cost impact would likely not be felt immediately. Instead, such costs would need to go through a review process in all provinces and be approved by the energy regulatory board of that respective province. Tight reserve margins can constrain electricity supply during extreme temperature events, amplifying short-term price volatility.

Consumer prices or "rates" vary for several reasons:

In many jurisdictions, the total electricity cost combines generation, transmission, distribution, and rider charges that appear separately on bills.

• variation in generation costs; prices in hydro-based provinces tend to be lower (e.g., British Columbia, Manitoba and Quebec);
• customer class (e.g., residential, commercial, industrial);
• residential rates tend to be higher than commercial and industrial rates;
• amount of energy consumed in a given period (e.g., rates may vary based on customer consumption);
• time-of-use pricing (e.g., some consumers have access to time of use meters that show lower charges for electricity during off peak periods);
• and capability to switch to lower cost fuels (e.g. industrial customers)

Consumers in deregulated provinces can compare plans from an electricity supplier to align contract terms and risk with their usage profile.

Electricity demand Canada in peak periods typically occurs during the winter months, although, at times, Ontario and Quebec experience peak electricity demand in the summer months due to increased use of air conditioning units. Spring and fall tend to be shoulder seasons with lower demand. Seasonal patterns also influence maintenance schedules for electricity generation across provinces, which can tighten supply during cold snaps or heat waves.

Related Articles

• Electricity Fundamentals

• Electricity Questions

• Electricity History

Definition of a rectifier explains AC to DC conversion using diodes, bridge or full-wave circuits, filters, and regulators to supply stable DC, reduce ripple, and power electronics, chargers, and DC motor drives.

What Is the Definition of a Rectifier?

An explanation of devices that convert AC to DC using diodes and filtering to deliver regulated, low-ripple DC power.

✅ Converts alternating current to direct current via diodes

✅ Topologies: half-wave, full-wave, bridge rectifier

✅ Uses filters and regulators to minimize ripple and stabilize DC

Understanding the definition of a rectifier is fundamental to grasping the complexities of power electronics and electrical engineering. Rectifiers are pivotal in converting AC power to DC power, a critical process for operating numerous electronic devices. By delving into the intricacies of half-wave, full-wave, and bridge rectifiers, as well as the silicon-controlled rectifier, it becomes clear that these devices are crucial in shaping the modern world of electronics. For readers new to the basics, an overview of the difference between AC and DC can clarify foundational concepts.

In electrical engineering, the definition encompasses a vital device that plays a crucial role in converting alternating current (AC) into direct current (DC). It  is an essential component in power electronics. It facilitates the conversion of AC power, commonly used in households and businesses, to DC power that electronic devices rely on. To contextualize this conversion, understanding alternating current behavior in typical distribution systems explains the need for rectification.

They come in various forms, such as diodes, mercury arc valves, and silicon-controlled rectifiers (SCRs). A diode, the most basic rectifying component, allows current to flow in only one direction. This feature helps convert AC power, which alternates direction, to DC power that flows in a single, continuous direction. For measurement and notation conventions, see how DC current is defined and applied in circuit analysis.

AC to DC conversion regulates voltage to create a steady and reliable energy supply. Throughout this conversion, rectifiers often assume different configurations depending on the specific application or requirement. Two popular configurations are the half-wave rectifier and the full-wave rectifier. Downstream stabilization is often handled by a voltage regulator that smooths residual ripple and safeguards loads.

A half-wave type allows only one half of the AC waveform to pass through while blocking the other half. This type is relatively simple and cost-effective but has a few downsides, such as lower efficiency and increased ripple voltage. Ripple voltage refers to the residual periodic variation of the DC output voltage. The result is an unsteady DC output, which might be undesirable in certain applications.

On the other hand, the full-wave type allows both halves of the AC waveform to pass through, effectively doubling the output frequency and reducing the ripple voltage. This configuration improves efficiency and provides a smoother, more consistent DC output voltage. The full-wave type has two primary forms: the center-tap configuration and the bridge rectifier.

The bridge rectifier is a highly efficient configuration that uses four diodes in a specific arrangement. This configuration eliminates the need for a center-tapped transformer, a key component in center-tap full-wave rectifiers, thus reducing overall size and cost. In addition, the bridge rectifier provides better voltage regulation and reduced harmonic distortion, making it ideal for various applications. For consistent terminology across datasheets, reviewing key electricity terms can prevent misinterpretation during design.

They serve numerous purposes in various fields. For instance, they are used in power supply circuits of electronic devices, battery chargers, and welding equipment. In addition, they help in voltage regulation for DC power supplies and extract radio signals from transmitted modulated signals. Many of these uses depend on stable direct current characteristics to ensure predictable device behavior.

Silicon-controlled rectifiers (SCRs) represent a more advanced type of rectifier. As silicon-based semiconductor switches, SCRs can control large amounts of current with a small input signal. This ability to manage current flow in a controlled manner sets SCRs apart from regular rectifiers, like diodes. As a result, SCRs are often used in power control applications, such as motor speed controllers, light dimmers, and temperature regulators.

Understanding how rectifiers play a pivotal role in industries and everyday applications is essential. One such application is in electric vehicles (EVs), where rectifiers contribute to charging systems by converting AC power from the grid to DC power for charging the battery. Additionally, they are utilized in renewable energy systems, like solar power and wind power, to convert the harvested energy into a stable and usable form for various applications. At a broader level, a concise electricity definition helps frame how conversion stages integrate with generation, storage, and loads.

Another application is in telecommunications equipment, where they help maintain a continuous and stable power supply. In the aviation and marine industries, rectifiers ensure sensitive equipment operates with minimal interference from fluctuating power sources. Moreover, they are employed in power supply units of medical devices, computers, and other critical equipment that demand stable power supplies.

How does it work in AC to DC conversion?

Its primary function is to convert AC voltage, which periodically reverses direction, into DC voltage, which flows in one direction. Its simplest form it consists of a diode, which allows current to pass in only one direction. When connected to an AC power source, the diode rectifies the current by blocking the negative half-cycles of the AC waveform. More advanced configurations, like full-wave rectifiers and bridge rectifiers, rectify both the positive and negative half-cycles, increasing efficiency and providing a smoother output.

What are the differences between half-wave and full-wave rectifiers?

The key difference between half-wave and full-wave rectifiers lies in their efficiency and output. A half-wave type only allows one-half of the AC waveform to pass through, blocking the other half. As a result, it has lower efficiency, increased ripple voltage, and produces an unsteady DC output.

On the other hand, a full-wave type allows both halves of the AC waveform to pass through, leading to increased efficiency and reduced ripple voltage. In addition, the full-wave type produces a smoother, more consistent DC output voltage, making it more suitable for applications that require a stable power supply. The full-wave type can be implemented using a center-tap or bridge rectifier configuration.

Related Articles

• Electricity Fundamentals

• Electricity Questions

• Electricity History