Biot Savart Law

Biot Savart Law and the study of electromagnetism have always been fascinating field of science that deals with the interaction between electric and magnetic fields. One of the cornerstones in this domain is this law, named after the French scientists Jean-Baptiste Biot and Félix Savart. This law plays a vital role in understanding the behaviour of magnetic fields produced by electrical currents.

Delving deeper into this fascinating concept, it describes the magnetic field generated by a tiny current flowing through a conductor. The law states that the magnetic field at a point is directly proportional to the current and inversely proportional to the square of the distance between the point and the current-carrying conductor. Furthermore, the magnetic field's direction is perpendicular to the plane formed by the unit vector and the straight wire carrying the current.

Biot Savart Law Explained

It is a fundamental principle in electromagnetism that describes the magnetic field produced by a steady electric current. It is named after French physicists Jean-Baptiste Biot and Félix Savart, who formulated the law in 1820. It provides a means to calculate the magnetic field at any point in space due to a specific current distribution.

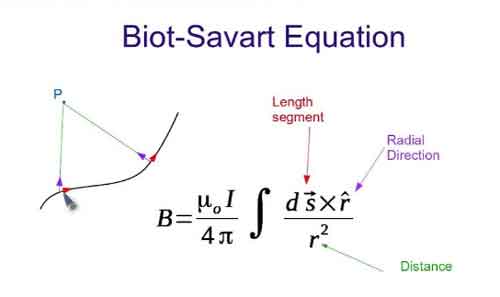
In mathematical terms, it can be expressed as:

dB = (μ₀ / 4π) * (Idl × r̂) / r²

Here,

dB represents the tiny magnetic field vector produced by an infinitesimal current element (Idl),
μ₀ is the permeability of free space (a constant with a value of 4π × 10^(-7) Tm/A),
I is the current flowing through the current element,
dl is the infinitesimal length vector of the current element,
r is the distance between the current element and the point in space where the magnetic field is being calculated,
r̂ is the unit vector pointing from the current element to the point of interest.

It states that the magnetic field produced by a small segment of a current-carrying conductor is proportional to the current, inversely proportional to the square of the distance from the point of interest, and depends on the angle between the current segment and the line connecting the segment to the point of interest.

To find the total magnetic field at a point in space due to an entire current distribution, you need to integrate the magnetic field contributions from each infinitesimal current element over the entire current-carrying conductor:

B = ∫dB

It is particularly useful for calculating the magnetic field in cases with complex current configurations or where symmetry is not apparent. In addition, it is often applied in situations where Ampere's Law would be difficult or impossible to use. Common applications include calculating magnetic fields due to straight wires, loops, and solenoids.

Maxwell's Equations

One might wonder about the relationship between the Biot Savart Law and Maxwell's Equations, a set of fundamental equations that govern the behaviour of electric and magnetic fields. It can be derived from Maxwell's Equations, specifically Ampere's Law, which establishes a connection between an electric current and the magnetic field it generates.

While the Biot Savart Law and Ampere's Law address magnetic fields, their focus and applicability differ. For example, the Biot Savart Law is more useful for calculating magnetic fields due to current loops or irregular current-carrying conductors. In contrast, Ampere's Law best suits symmetrical geometries and steady-state currents.

The practical applications of the Biot Savart Law are abundant, ranging from designing and analyzing electromagnets and generators to determining the magnetic moment of a current loop, an essential parameter in magnetostatics. The law has also significantly developed technologies like magnetic resonance imaging (MRI), particle accelerators, and magnetic storage devices.

The versatility of the Biot Savart Law is exemplified by its ability to calculate magnetic fields of various geometries. From a straight wire or a solenoid to more complex configurations like toroidal or saddle-shaped coils, this law is a reliable tool for understanding the behaviour of magnetic fields in different scenarios.

Delving into the connections between different laws, the relationship between the Biot Savart Law and Faraday's Law is quite intriguing. While the Biot Savart Law is concerned with the magnetic field generated by a current, Faraday's Law deals with the electromotive force (EMF) induced in a conductor due to a changing magnetic field. Together, these laws form the basis of electromagnetic induction, which underpins the functioning of electric generators, transformers, and inductors.

The study of electromagnetism would only be complete by touching upon the concept of electric fields. Like magnetic fields, electric fields are fundamental aspects of electromagnetism, originating from electric charges. Coulomb's Law governs the interaction between electric charges, drawing parallels with the Biot Savart Law, which deals with magnetic fields generated by electric currents.

In the realm of vector calculus, the Biot Savart Law uses the cross product and the dot product to define the magnitude and direction of the magnetic field. These mathematical tools enable scientists and engineers to model and analyze the behaviour of magnetic fields in various applications.

One of the key parameters in the Biot Savart Law is the permeability of free space, denoted by the symbol μ₀. This constant defines the ease with which a magnetic field can permeate through the vacuum and is crucial in determining the strength and reach of the magnetic field generated by a current.

It is an essential concept in the study of electromagnetism. It provides a foundation for understanding magnetic fields generated by electric currents and connects with other fundamental laws, such as Ampere's Law, Faraday's Law, and Coulomb's Law. Moreover, the diverse applications of the Biot Savart Law, ranging from designing electromagnets to analyzing complex magnetic field geometries, showcase its versatility and importance.

As we continue to explore the world of electromagnetism, the Biot Savart Law will remain a crucial tool in our arsenal. Through rigorous research and innovative technologies, scientists and engineers will continue to unlock the mysteries of electric and magnetic fields, ultimately leading to groundbreaking discoveries and advancements that have the potential to transform our world.

From powering our homes with electricity to enabling cutting-edge technologies like MRI machines and particle accelerators, it plays a central role in shaping the science and engineering landscape. As we progress into the future, this law will undoubtedly continue to serve as a beacon of knowledge and understanding in the ever-evolving realm of electromagnetism.